Lecture 2 - Chemical Kinetics (Part 2)

Lecture Recording:



Lecture Summary/Notes:
Hi guys!! So I'm trying out spoilers! Just click the lines that look like this to expand or minimize the notes for the related topic. I think this will make things easier to navigate through (and it looks more organized, yay!). I'm also scanning my notes for diagrams and math this time. It's a bit more messy than doing it with the computer, I hope you don't mind :) - they ARE more colorful, though :).

Second Order Reactions

We started the lecture with the continuation of chemical kinetics, picking up with second order reactions. For a generic reaction A -> Products, the rate law R=k[A]^2 was integrated (to make it into y=mx +b) form. Here it is:

If experimentally found data points were graphed, and the reaction was second order, it would look like this graph (a straight positive slope)~

Pseudo First Order Conditions

This is used to trick the system into thinking a second order reaction is an overall first order  reaction. For example, for the generic reaction A+B -> Products, you start the reaction with highly disproportionate amounts of A (0.01mol) and B (1.00mol). When the reaction proceeds, A will change a LOT (the reaction uses 100% of its initial amount), and B will change minimally (the change is from 1.00 to 0.99). Therefore, B's contribution to the reaction rate is minuscule, and becomes a constant, making the rate law: R = K'[A], where K'= K[B]. This way, the reaction becomes a first order reaction, and can be used to verify whether A is really a first order reactant or not. This can be verified by graphing  experimentally found data points to see if it matches the corresponding graph. These same steps can be used for B.

If data entered produces a curved line, it means that the reaction does not match the order.

Activation Energy

These graphs show the energy of a reaction:

Reaction Coordinate - An imaginary concept of how far along the reaction is.

At the top of each 'hill' (highlighted in pink) is a transition state. At this point, if it were a reaction A+B->C, there is some collision of A and B (there may be other things in there too, we don't really know). Anyways, what's important is that the activation energy is the energy of this state.


The rate of the reaction depends on the activation energy. As Ea increases, the speed of the reaction increases (this is because if there is a high Ea, the reaction must climb a steeper hill, making it slower).


How the reaction rate is related to the activation energy (mathematically) is shown by the Arrhenius Equation.

Arrhenius Equation

This equation relates K (the rate constant) to Ea. A (circled in pink) is the pre-exponential factor. It is related to how fast the molecules get to each other (the fastest rate you can possibly get for that reaction.

• As T approaches infinity, K approaches A
• Increasing temperature speeds up the reaction
• As T approaches 0 Kelvins, K approaches 0. 
• Lowering temperature slows the speed of reaction

We then proceeded to rearrange the equation so that it would be in the y=mx+b format:

The Arrhenius plot can be used to find the Ea of the transition structure.
It gives us hints about the mechanisms involved.
There are sections of the graph that are extrapolated since you cannot have 0 Kelvins nor infinite temperature.

Next, we can put the Arrhenius equation into a very convenient format, one familiar to us thanks to the Clausius-Clapeyron equation:

Mechanisms

Be warned. This topic is very long!


What is a mechanism?
It is a step by step sequence of elementary processes (i.e. 1 molecule A + 1 molecule B = 1 molecule C).


When given reaction rates, you can propose mechanisms (guess what steps are taken to get the overall reaction). The following are some guidelines for making proposed mechanisms:

• The proposed mechanism must be possible (molecules are colliding randomly)!
• 4 molecules colliding at once - impossible
• 3 molecules colliding at once - highly improbable
• 2 molecules colliding at once - very likely
• The proposed mechanism must be fitting for its activation energy!
• A harder molecule to form will result in a higher Ea
• i.e. molecules that break the octet rule
• An easier-to-form molecule will result in a low Ea.
• Any proposed mechanism will have a unique rate law equation
• But if given a rate law, there may be many possible mechanisms

Hypothetical Example:

Proposed mechanism for the overall reaction  A+B->C+D:

1. 2A->X
• Two molecules of A gives some species X
2. X+B->Y+A+C
• X collides with B, producing Y, A, and C
3. Y->D
• Y is an unstable species, and thus decomposes, forming D
• Let's say this reaction has the slowest rate

Rate Determining Step:

One of these steps are going to be slower than the others, which is usually the case in sequenced mechanisms. Also, reactions can only be as fast as their slowest step. This is called the rate determining step. You write the rate law based only on the rate determining step, and then rearrange it so that it relates only the reactants of the original (general) equation. 

Real Example

Proposed mechanism for the overall reaction H2+2ICl -> I2+2HCl based on the  experimentally determined rate law R= K[H2][ICl]:

1. H2 + ICl -> HI + HCl
2. HI + ICl -> I2 + HCl

*Identify what step you think is the slow step*

(It's step 1 - you need to dissociate the H2 gas, which does not happen very quickly compared to HI, which is a good acid)

*Write the rate law equation based on the rate determining step*

( R = K [H2] [ICl] )

*Rearrange the rate law equation to match the reactants of the overall equation*

(It is already written in terms of the overall reactants)

If we were to graph the energy of this reaction, we would get a graph like this:

There are two transition states, and one intermediate, which in this case is HI.

Real Example #2

We went through two possible mechanisms, and rejected the first for the noted reason.
We chose the second step of the second mechanism to be the rate determining step since we know that the first step involves a fast equilibrium, and then wrote out the rate law for the second step. We do encounter a problem - it is written related to an intermediate, as is shown in the following:

Note that there are separate k values for each  elementary step, and two k values for step 1. The next scan shows the rearrangement used to isolate the intermediate. For the equilibrium step, the rate of the forward reaction is equal to the rate of the reverse reaction. Also, note that the equilibrium constant is basically the overall rate constant of step 1.

At the end, the proposed rate law matches that of the experimental... VICTORY!

Experiments for Measuring Reaction Rates

We covered two different experiments used to measure reaction rates.

Steady State Condition

The steady state approximation is used to help determine the rate determining step. This can only be used if there is an intermediate!


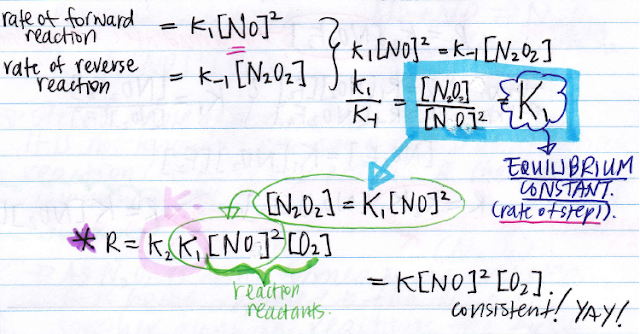
Let's use an example to explain. The overall reaction is: 2NO +O2 -> 2NO2. The experimental rate law is - R= k[NO]^2[O2]. The proposed mechanism is:

Where N2O2 is the intermediate.

The general theory behind steady state condition is this: as N2O2 is being formed in the first step, it is being decomposed in the second step. Further along the reactions, the first step slows down (due to the decrease in reagents) and the second speeds up (due to more abundant reagents). At some point in the mechanism, the two steps have the SAME rate - the concentration change in N2O2 is 0 - it reaches a steady state. 


First, we'll guess the slow step based on the experimental rate law. We will guess it to be the second step (N2O2 + O2  -> 2NO2 ) since there is an O2 in this step, which matches that of the experimental rate law. Now, we will use the steady state approximation to verify this guess. 


Set the rate of formation of N2O2 to be the same as the rate of decomposition of N2O2:


**Scan of this is coming**


Then solve for N2O2. 


**Scan of this is coming**

Catalysts

Catalysts speed up the rate of reaction (i.e. enzymes - you have millions of these in your body catalyzing millions of reactions). 

There are two classifications of catalysts:

1. Homogeneous
• These catalysts are dissolved in the solution with the reagents (they co-dissolve)
• Enzymes are homogeneous catalysts
2. Heterogeneous
• These catalysts exist in different stages from the reagents
• Reactions that take place on the surface are heterogeneous
• For example, when reagents are in the gas phase, and the catalyst is solid, the catalyst is heterogeneous
• The reaction takes place on the surface of the solid.
• These are usually zero order reactions

Textbook Examples Used in Class