Intro :)

Hello! For anyone reading, welcome! I've completed the course, so I won't be posting any more updates. I will, however leave the material for anyone else who finds it and reply to any questions/comments.

Dr. Pietro taught this course, and the lecture recordings are those of him teaching it :)

The purpose of this blog was to provide help and resources for my classmates in the CHEM1001 course. Over the span of approximately 3 months that this blog has been up, it's been visited 4500+ times, excluding my own pageviews. Hopefully, that means my 200+ classmates found this blog helpful :)

I've changed the dates in order to reorder the posts for future chem1001 students. 
Note that the corresponding textbook for this course was Pearson - General Chemistry: Principles and Modern Applications (10th edition).

-Joy

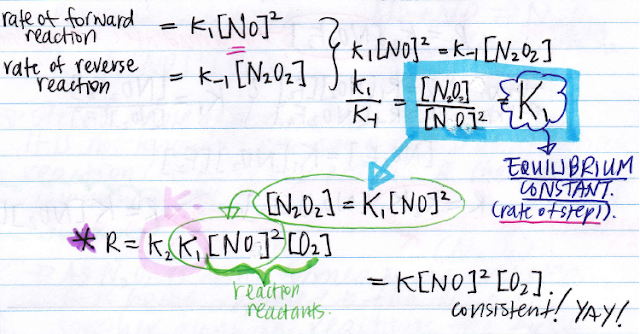
------Original Post------


Hey everyone! I'm here with my first post! I'm still figuring things out, so if you have any suggestions or comments (either now or later) please contribute with a comment :). 


Basically, I'm just here to provide you guys with a ton of help that you may or may not need. I'm going to be putting up lecture recordings and lecture notes/summaries


With regard to the recordings, they're not really intended to replace going to lectures! I personally use them to fill in missing bits in my notes, and to try to get a better understanding of the material (it seems to come through the second time around). Also, since our professor uses the board while explaining things, it might be difficult to follow along unless you've got the visuals written down... Maybe I'll put up my notes as well...ANYWAYS, go to class! You'll find me sitting front and center if ever you want to say hi :P.


I hope many of you will find this blog helpful! Let's ace chemistry~~~ YAYY!!!


-Joy

Lecture 1 - Chemical Kinetics!

Lecture Recording:

Lecture Summary/Notes:

OK, so basically, we started this course with chemical kinetics! 

Key Questions covered in the lecture:

-What makes a reaction fast or slow?

-How do we measure or define that (the rate of the reaction)?

Kinetics, unlike thermochemistry, is very UNPREDICTABLE!

To explain this - if you look at a curved function, at every point, the tangent is a different slope, right? The energy of a reaction (E)  is like a curved function, with a rising hill, and then a drop, like this! 

Initially, you have the reactants (left side), then the reactants come together to form something, then they break apart to make the products (right side). At every point in this reaction, the rate is different! The point where the red star is, that's called the transition state! Something is going on there, but we don't know what! But what we DO know, is that if there are 4 reactants, A, B, C, and D, there is NO chance that they are all going to collide one another at the same time to produce the product. This point hints at the mechanism involved in the reaction.

How do we define the rate of the reaction?
**We must define the conditions first (since they are always changing!)


WAYS to define the rate:
Average rate (change in concentration over the change in time). 
Initial rate (slope of the tangent at x=0). 
Both the average rate and the initial rate are not very accurate ways to measure rate... Initial rate depends on the initial conditions of the reaction!


SO WHAT DO WE USE????
The rate equation (or the definition of a rate)


The overall order of a reaction is the SUM of all the orders.
The order is usually a very small integer or simple fraction!




The Zero Order Reaction (where m = 0 in R= k[A]^m)
Looks like this!

Enzyme catalyzed reactions are usually zero-order in some species!

The rate equation for the zero order equation goes something like this:

R= k[A]^0

R=k

Slope m (from y=mx+b) is -k (since the reactant is disappearing)
[A]t = -kt + [A]

(the concentration of A at any time t = -kt + initial amount of reactant)

At this point, we started talking about Radioactive Decay, which is always a first order reaction! (first order reactions are the most common in nature). That brought us somehow to the topic of the integrated rate law. 

The Rate Law was integrated in order to isolate [A], which tells us the concentration of A at any given time :).
We can also use the final result to determine HALF-LIFE,  which is just found by setting [A]t = [A]0/2

Reactions Involving Gas

Conclusion:
Kinetics hint to and give us information about the MECHANISMS of a reaction!

Lecture 2 - Chemical Kinetics (Part 2)

Lecture Recording:



Lecture Summary/Notes:
Hi guys!! So I'm trying out spoilers! Just click the lines that look like this to expand or minimize the notes for the related topic. I think this will make things easier to navigate through (and it looks more organized, yay!). I'm also scanning my notes for diagrams and math this time. It's a bit more messy than doing it with the computer, I hope you don't mind :) - they ARE more colorful, though :).

Second Order Reactions

We started the lecture with the continuation of chemical kinetics, picking up with second order reactions. For a generic reaction A -> Products, the rate law R=k[A]^2 was integrated (to make it into y=mx +b) form. Here it is:

If experimentally found data points were graphed, and the reaction was second order, it would look like this graph (a straight positive slope)~

Pseudo First Order Conditions

This is used to trick the system into thinking a second order reaction is an overall first order  reaction. For example, for the generic reaction A+B -> Products, you start the reaction with highly disproportionate amounts of A (0.01mol) and B (1.00mol). When the reaction proceeds, A will change a LOT (the reaction uses 100% of its initial amount), and B will change minimally (the change is from 1.00 to 0.99). Therefore, B's contribution to the reaction rate is minuscule, and becomes a constant, making the rate law: R = K'[A], where K'= K[B]. This way, the reaction becomes a first order reaction, and can be used to verify whether A is really a first order reactant or not. This can be verified by graphing  experimentally found data points to see if it matches the corresponding graph. These same steps can be used for B.

If data entered produces a curved line, it means that the reaction does not match the order.

Activation Energy

These graphs show the energy of a reaction:

Reaction Coordinate - An imaginary concept of how far along the reaction is.

At the top of each 'hill' (highlighted in pink) is a transition state. At this point, if it were a reaction A+B->C, there is some collision of A and B (there may be other things in there too, we don't really know). Anyways, what's important is that the activation energy is the energy of this state.


The rate of the reaction depends on the activation energy. As Ea increases, the speed of the reaction increases (this is because if there is a high Ea, the reaction must climb a steeper hill, making it slower).


How the reaction rate is related to the activation energy (mathematically) is shown by the Arrhenius Equation.

Arrhenius Equation

This equation relates K (the rate constant) to Ea. A (circled in pink) is the pre-exponential factor. It is related to how fast the molecules get to each other (the fastest rate you can possibly get for that reaction.

• As T approaches infinity, K approaches A
• Increasing temperature speeds up the reaction
• As T approaches 0 Kelvins, K approaches 0. 
• Lowering temperature slows the speed of reaction

We then proceeded to rearrange the equation so that it would be in the y=mx+b format:

The Arrhenius plot can be used to find the Ea of the transition structure.
It gives us hints about the mechanisms involved.
There are sections of the graph that are extrapolated since you cannot have 0 Kelvins nor infinite temperature.

Next, we can put the Arrhenius equation into a very convenient format, one familiar to us thanks to the Clausius-Clapeyron equation:

Mechanisms

Be warned. This topic is very long!


What is a mechanism?
It is a step by step sequence of elementary processes (i.e. 1 molecule A + 1 molecule B = 1 molecule C).


When given reaction rates, you can propose mechanisms (guess what steps are taken to get the overall reaction). The following are some guidelines for making proposed mechanisms:

• The proposed mechanism must be possible (molecules are colliding randomly)!
• 4 molecules colliding at once - impossible
• 3 molecules colliding at once - highly improbable
• 2 molecules colliding at once - very likely
• The proposed mechanism must be fitting for its activation energy!
• A harder molecule to form will result in a higher Ea
• i.e. molecules that break the octet rule
• An easier-to-form molecule will result in a low Ea.
• Any proposed mechanism will have a unique rate law equation
• But if given a rate law, there may be many possible mechanisms

Hypothetical Example:

Proposed mechanism for the overall reaction  A+B->C+D:

1. 2A->X
• Two molecules of A gives some species X
2. X+B->Y+A+C
• X collides with B, producing Y, A, and C
3. Y->D
• Y is an unstable species, and thus decomposes, forming D
• Let's say this reaction has the slowest rate

Rate Determining Step:

One of these steps are going to be slower than the others, which is usually the case in sequenced mechanisms. Also, reactions can only be as fast as their slowest step. This is called the rate determining step. You write the rate law based only on the rate determining step, and then rearrange it so that it relates only the reactants of the original (general) equation. 

Real Example

Proposed mechanism for the overall reaction H2+2ICl -> I2+2HCl based on the  experimentally determined rate law R= K[H2][ICl]:

1. H2 + ICl -> HI + HCl
2. HI + ICl -> I2 + HCl

*Identify what step you think is the slow step*

(It's step 1 - you need to dissociate the H2 gas, which does not happen very quickly compared to HI, which is a good acid)

*Write the rate law equation based on the rate determining step*

( R = K [H2] [ICl] )

*Rearrange the rate law equation to match the reactants of the overall equation*

(It is already written in terms of the overall reactants)

If we were to graph the energy of this reaction, we would get a graph like this:

There are two transition states, and one intermediate, which in this case is HI.

Real Example #2

We went through two possible mechanisms, and rejected the first for the noted reason.
We chose the second step of the second mechanism to be the rate determining step since we know that the first step involves a fast equilibrium, and then wrote out the rate law for the second step. We do encounter a problem - it is written related to an intermediate, as is shown in the following:

Note that there are separate k values for each  elementary step, and two k values for step 1. The next scan shows the rearrangement used to isolate the intermediate. For the equilibrium step, the rate of the forward reaction is equal to the rate of the reverse reaction. Also, note that the equilibrium constant is basically the overall rate constant of step 1.

At the end, the proposed rate law matches that of the experimental... VICTORY!

Experiments for Measuring Reaction Rates

We covered two different experiments used to measure reaction rates.

Steady State Condition

The steady state approximation is used to help determine the rate determining step. This can only be used if there is an intermediate!


Let's use an example to explain. The overall reaction is: 2NO +O2 -> 2NO2. The experimental rate law is - R= k[NO]^2[O2]. The proposed mechanism is:

Where N2O2 is the intermediate.

The general theory behind steady state condition is this: as N2O2 is being formed in the first step, it is being decomposed in the second step. Further along the reactions, the first step slows down (due to the decrease in reagents) and the second speeds up (due to more abundant reagents). At some point in the mechanism, the two steps have the SAME rate - the concentration change in N2O2 is 0 - it reaches a steady state. 


First, we'll guess the slow step based on the experimental rate law. We will guess it to be the second step (N2O2 + O2  -> 2NO2 ) since there is an O2 in this step, which matches that of the experimental rate law. Now, we will use the steady state approximation to verify this guess. 


Set the rate of formation of N2O2 to be the same as the rate of decomposition of N2O2:


**Scan of this is coming**


Then solve for N2O2. 


**Scan of this is coming**

Catalysts

Catalysts speed up the rate of reaction (i.e. enzymes - you have millions of these in your body catalyzing millions of reactions). 

There are two classifications of catalysts:

1. Homogeneous
• These catalysts are dissolved in the solution with the reagents (they co-dissolve)
• Enzymes are homogeneous catalysts
2. Heterogeneous
• These catalysts exist in different stages from the reagents
• Reactions that take place on the surface are heterogeneous
• For example, when reagents are in the gas phase, and the catalyst is solid, the catalyst is heterogeneous
• The reaction takes place on the surface of the solid.
• These are usually zero order reactions

Textbook Examples Used in Class

Lecture 3 - Chemical Kinetics (Part 3) and Chemical Equilibrium!

Lecture Recording:


Lecture Summary/Notes:

We finished off chemical kinetics today, with enzyme-substrate reactions and started chemical equilibrium. Again, just click the lines that look like this to expand or minimize the notes for the related topic.

Enzyme-Substrate Reactions

All enzymes have the ability to react/work on one substrate. We talked about the mechanism and the rate of this reaction:

Enzyme-substrate reactions is a 2-step process. The enzyme and the substrate come together to form an enzyme-substrate complex (the intermediate). The intermediate then decomposes to leave the enzyme and some product. *Assume that ES is in steady state (it is being formed at the same rate that it is being consumed - refer to lecture 2 for more on steady state conditions).

So, since we assumed that the second step is the rate determining (slow) step, we use it to form the rate law (this is the purpose of everything that follows - to find the rate law!). The second line is using the steady state principle: ES is being formed at the same rate in which ES is being consumed (in the reverse step of the equilibrium and in the second step). We factor out the [ES].
The third line is saying that the concentration of the enzyme + the concentration of ES is equal to the initial concentration of enzymes. We can rearrange this line to say [E] = [E]0-[ES]. We substitute this into the second line (steady state equation), and we get:

The second line here is what we get when we foil out the first line, multiply it by [S], and rearrange it a bit. (So there should be one line in between the first and the second that goes  K1[E]0[S] - K1[ES][S] = [ES](K-1 + K2). Then in the third line, we factor out the [ES], then we isolate [ES]. We THEN sub [ES] back into the FIRST equation (the rate law) to get the rate. We did all this because we cannot have the rate law in terms of the intermediate.

Alright, now that we have the rate, we used Km to lump the K constants together, so that we can simplify the math a little bit. Then, we set two conditions (what could happen). If Km is a lot larger than [S], then the reaction will be first order for the substrate. Vice-versa, if [S] is a lot larger than Km, then the reaction will be zero-order for the substrate. This explains why enzyme-substrate reactions are sometimes first order, and at other times zero order.

Chemical Equilibrium - Introduction

We started  chemical equilibrium with an example which went something like this: imagine that all the girls in the class started at the right side of the classroom and all the boys on the left. Then, the whole class was blindfolded and told to run around in any direction (everyone had the ability to walk/run straight and bounce off walls). After a certain time, there would be roughly an equal number of girls and boys on each side of the room (there will be some running to the other side and others bouncing off of walls heading to the other side). After that time, there will be a dynamic equilibrium, which is where the number of students of each gender on either side does not change, but everyone is still moving. The following is a graph representing this example:


So if we put this example into an equation, there is an equilibrium of the concentration of either boys or girls the right side [A] and the left side [B] (there are as many people going to the left side as there are going to the 
 
We find the rate law for both the people going from A to B (forward reaction), and for those going from B to A (reverse reaction). We will say that the rate constant for the forward reaction is K1 and the rate constant for the reverse reaction is K-1. The rates are equal, so we say that K1[A] = K-1[B]. We use this to find the equilibrium constant which is [B]/[A]

This example shows a general way to find the equilibrium constant of any aqueous equilibrium reaction (concentrations of the products to the power of their coefficients over the reactants to the power of their coefficients). Also, it shows that you can get an equilibrium constant from overall reactions, though you cannot get rates. However, the concentrations are just a part of what makes up ACTIVITY, which is what really makes up the equilibrium constant.

Activity

The following shows an example of finding the equilibrium constant using the method learned above, and what it really represents:

Just in case you can't read my writing... I wrote that the concentrations are REALLY activities... not just concentrations! So the activity of the products, raised to the power of their coefficients over the reactants to their powers are what make up K. Activity is unitless and ranges from 0-1. For aqueous solutions, activity can be found by using just the concentrations, but as we will learn later on, it is not the case for gaseous or pure liquid/solid states.

As a solution becomes more dilute, the activity coefficient approaches 1. This is why we can use just the concentrations when finding the activity of aqueous solutions.  

Pretty straight forward. Except for the last rule, when finding the activity for a gas, you can just say that activity of a gas = the partial pressure of that gas (in atm).

An example involving gases.

*See example 1 in the textbook examples section

Manipulation of Equations

• If you have multiple equations that add up resulting in an overall equation, the equilibrium constant of an overall equation = the product of individual equilibrium constants.

• If you multiply an equation through by a constant, you raise the equilibrium constant to the power of that constant (e.g.  2 times A+B <=> C+D, K^2)

• If you reverse an equation, you take the reciprocal of the equilibrium constant (e.g. C+D<=> A+B, 1/K)

*See example 2 in the textbook examples section

Gases (Equilibrium Constant)

The following is a generalization of how to find the equilibrium constant for equations involving gases (using partial pressures).

We then sought a way to find the equilibrium constant for equations involving gases with concentrations, as we did before.

Hopefully this is all pretty straight forward, and is easy to follow along. Remember that delta n involves only the coefficients of gas molecules!

*See example 3 in the textbook examples section

Textbook Examples Used in Class

Example 1 - Find the concentration of [Hg2]2+ using the given concentrations and the equilibrium constant.

Example 2 - find the equilibrium constant of the second equation given the first equation and its equilibrium constant.

Example 3 - Find the pressure-based equilibrium constant of the given equation given the concentration-based equilibrium constant and the temperature.

Example 4 - Find the concentration based equilibrium constant of the given equation (remember that pure liquids and solids have an activity of 1).

Example 5 - Find the pressure-based and concentration-based equilibrium constants of the given equation.